Fig. No. 3 shows the electron microscope image of the fracture surface of a spherical foam glass sample made of sintered coloured waste glass powder (0≤Φ≤125μ), whose density is ρ=1.75gr / cm3 . During sintering the maximum temperature is held for 11 minutes at 960°C.
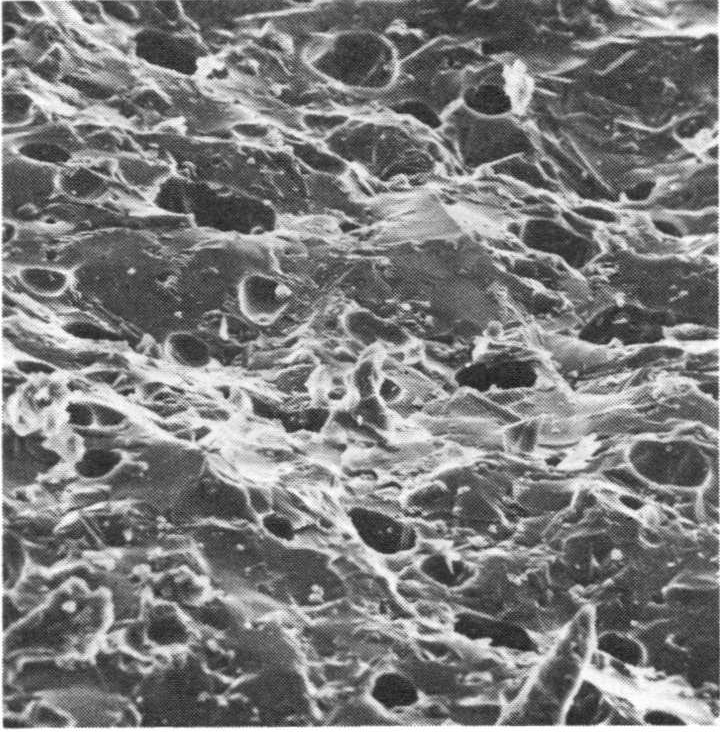
Fig No. 3. Fracture surface of a spherical foam glass sample at scale 392 : 1
Using X-ray investigation it was possible to determine that the sample under consideration here contained approximately 10 + 15 % devitrite and traces of cristobalite1) . Based on a thin microsection, the mean free distance of the spherical bubbles of the spherical foam glass sample shown here was determined at 80 μ .
An analysis of the section of the polyhedron foam glasses produced by us generally shows the structure represented in Fig. No. 4.

Fig No. 4 section of a coarse-celled polyhedron foam glass sample
1) SiO2. is polymorphous and for the reason occurs naturally in three crystalline forms:
- 1)as quartz
- 2)as cristobalite (cubic) and
- 3)as tridymite (hexagonal).
This is a section of the same foam glass cuboid as shown in Fig. No. 1. Here you can see the richness of forms of the innumerable polyhedron cell bubbles!
In order to express that the cell walls are comprised of fixed shape and low capillary space spherical foam glass, in future we will refer to them as films 1).
Images of microsections through a given polyhedron foam glass sample using polarised light show that the glass base material of the cell wall films contain many small embedded crystals as well as chemically unreacted expanding agent.
Since in the original glass melt - from which the polyhedron foam glass is expanded - surface tensions are active, the physical principles for liquid polyhedron foams, as described in section 1.2, can also be used for the solidified polyhedron foam glass. For this reason it can be asserted that in statistical mean the morphology of all given two-dimensional sections through a random polyhedron foam glass sample can be expressed using the following idealised sketch - in accordance with the principles of J. Plateau.
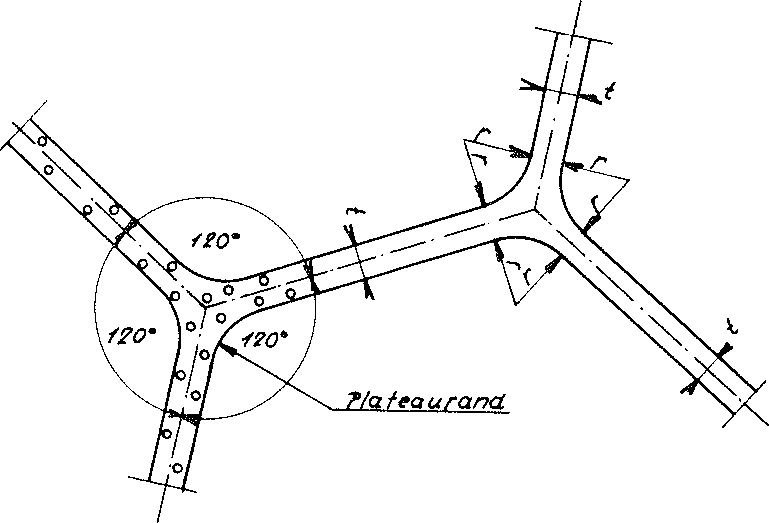
Idealised section through a polyhedron foam glass sample
- 1) E. Manegold understands a film to be a fixed-form low-capillary space sheet, whereas he understands a membrane to be a fixed-form high-capillary space sheet (1).
We also see that at points where three cell wall films normally meet with the equilibrium angle of 120°, the surfaces are rounded cylindrically with so-called plateau edges.